经济Final Exam代考 EC 399 Final Exam (Version B)

2021 Winter EC 399 Final Exam (Version B)

经济Final Exam代考  Suppose that player 1 has Rawsian social  preference.  That  is,  she  cares  about  the  mini- mum  payoff of the two  players.  Player

Problem 1 (20 pts) 经济Final Exam代考

Suppose Larry first win $3000 in a casino two days ago. Then he lost another $300 in the stock mar- ket x < 0. Assume that Larry’s value function is given by v(x) = x/3 when x ≥ 0; v(x) = −3√−x when x < 0.

• What is the total value of Larry if he integrates the gain and the loss?   What is the total       value of Larry if he separates the the loss from gain? From Larry’s view, should he integrate or separate? (10 pts)


• Please draw the graph that illustrates the values corresponding to Larry’s gain and loss and   the integration of the (10 pts)

Problem 2 (30pts) 

Assume that JB is going to watch  one movie.  He can go to Movie A in week 1, or go to Movie B   in week 2, or go to Movie C in week 3. Suppose Movie A gives him a utility of 3, Movie B gives him a utility of 5, Movie C gives him a utility of 8.

• Suppose that JB is an exponential discounter with parameter δ = 0. Which movie would he eventually watch? Try to depict the three movies on a graph and draw the different time indif- ference curves that go through them. (10 pts)

• Suppose that JB is a naive hyperbolic discounter with parameter δ = 0.9 and β = 0. Which movie would he eventually watch? (10 pts)

• Suppose that JB is a sophisticated hyperbolic discounter with parameter δ = 0.9 and β = 0. Which movie would he eventually watch? (10 pts)

Problem 3 (10pts)  经济Final Exam代考

Suppose Player 1 is a male player, player 2 is a female player.

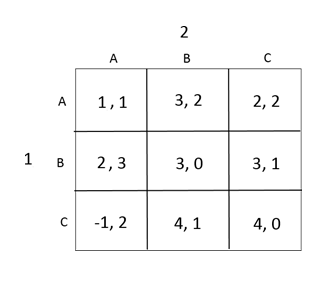
Assume that player 1 is a level-2 male player, player 2 is a level-4 female player. Suppose that in the real world, level-0 male player tends to choose B, level-0 female player tends to choose C. What is the prediction of the game? Explain.

Problem 4 (30pts)

• Suppose Joanne is presented with a box filled with 60 colored tickets. Joanne knows that 20 of them are black, 40 of them are either blue or green. Suppose Joanne is invited to randomly draw a ticket from the She is offered the following gambles: (A) if the ticket is black, she gets $10.

(B) If the ticket is green, she gets 10.  (C) If the ticket is either black or blue, she gets $10.  (D) If  the ticket is either blue or green, she gets $10.

Draw a table for the four gambles. Point out which column corresponds to “sure thing.” Suppose Joanne prefers (B) to (A) and she prefers (D) to (C). Does she violates the sure-thing principle?     (10 pts)

• Please provide an example of your own on preferences over (10 pts)

• Explain what probability weighting means. (10 pts)

Problem 5 (40pts) 经济Final Exam代考

• Consider the following game table. Numbers in the table represent monetary


• 

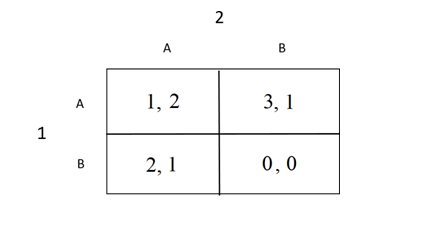
1a) Suppose both players only care about monetary payoffs. Find if any player has a strictly dom- inated strategy. What is the prediction of the game if both players never play strictly dominated strategies? (5 pts)

1b) Suppose that player 1 has Utilitarian preferences.   That is,  she cares about her own  mone-    tary payoff plus player 2’s monetary payoff. Player 2 has spiteful preferences with a spiteful level  of 1. That is, he cares about his own monetary payoff minus player 1’s monetary payoff. Draw the corresponding new game table, circle the best responses and find the pure Nash equilibria. (15pts)

• Cosider the following game tree. Numbers in the tree represent monetary

2a) Suppose both players only care about monetary payoffs. Find the subgame perfect equilibria. (5 pts)

2b) Suppose that player 1 has Rawsian social  preference.  That  is,  she  cares  about  the  mini- mum  payoff of the two  players.  Player 2 has altruistic preferences with a altruistic level of 0.5.  That is, he cares about his own monetary payoff plus half of player 1’s monetary payoff. Draw the corresponding new game tree, find the subgame perfect equilibria. (15 pts)

Problem 6 (20 pts)

Suppose Kristine’s value function is given by v(x) = √2x for gains (x ≥ 0) and v(x) = −√−3x for losses (x < 0). Kristine is facing the choice between option (A) a sure amount $140 and option (B)  a gamble which gives Kristine $200 with probability 0.6 and $50 with probability 0.4.

• If Kristine uses $0 as her reference point, what are the values  of the two  options?  Which   option would Kristine prefer? Draw the graph of Kristine’s value function, point out the values corresponding to the two options on the graph. (10 pts)

• If Kristine uses $140 as her reference point, what are the values of the two options?  Which  option would Kristine prefer? Draw the graph of Kristine’s value function, point out the values corresponding to the two options on the graph. (10 pts)

更多代写： HomeWork cs作业     金融代考    postgreSQL代写         IT assignment代写     统计代写 怎么修改英语essay