final考试代考_数学期末考试代考 Mathematics 220 Final Examination

Mathematics 220 Final Examination

数学期末考试代考 Each examination candidate must be prepared to produce, upon the request of the invigilator or examiner, his or her UBCcard for

Student Conduct during Examinations  数学期末考试代考

• Each examination candidate must be prepared to produce, upon the request of the invigilator or examiner, his or her UBCcard for identification.
• Candidates are not permitted to ask questions of the examiners or invigilators, except in cases of supposed errors or ambiguities in examination questions, illegible or missing material, or the like.
• No candidate shall be permitted to enter the examination room after the expiration of one-half hour from the scheduled starting time, or to leave during the first half hour of the examination. Should the examination run forty-five (45) minutes or less, no candidate shall be permitted to enter the examination room once the examination has begun.

• Candidates must conduct themselves honestly and in accordance with established rules for a given examination, which will be articulated by the examiner or invigilator prior to the examination commencing. Should dishonest behaviour be observed by the examiner(s) or invigilator(s), pleas of accident or forgetfulness shall not be received.

• Candidates suspected of any of the following, or any other similar practices, may be immediately dismissed from the examination by the examiner/invigilator, and may be subject to disciplinary action: 数学期末考试代考

(a) speaking or communicating with other candidates, unless otherwise authorized;
(b) purposely exposing written papers to the view of other candidates or imaging devices;
(c) purposely viewing the written papers of other candidates;
(d) using or having visible at the place of writing any books, papers or other memory aid devices other than those authorized by the examiner(s); and,
(e) using or operating electronic devices including but not limited to telephones, calculators, computers, or similar devices other than those authorized by the examiner(s)–(electronic devices other than those authorized by the examiner(s) must be completely powered down if present at the place of writing).

• Candidates must not destroy or damage any examination material, must hand in all examination papers, and must not take any examination material from the examination room without permission of the examiner or invigilator.
• Notwithstanding the above, for any mode of examination that does not fall into the traditional, paper-based method, examination candidates shall adhere to any special rules for conduct as established and articulated by the examiner.
• Candidates must follow any additional examination rules or directions communicated by the examiner(s) or invigilator(s).

Please read the following points carefully before starting to write. 数学期末考试代考

• Read all the questions carefully before starting to work.
• You should give complete arguments and explanations for all your answers and calculations; answers without justifications will not be marked.
• Continue on the back of the previous page if you run out of space.
• Attempt to answer all questions for partial credit.
• This is a closed-book examination. None of the following are allowed: documents, cheat sheets or electronic devices of any kind (including calculators, cell phones, etc.)
• You may not leave during the first 30 minutes or final 15 minutes of the exam.

1. For Part (a) to Part (g), determine whether the statements are true or false — Put True or False in the boxes. Part (h) is not a True/False type question. Justify your answers.

(a) (4 marks) Let A, B be sets. Then P(A ∪ B) ⊆ P(A) ∪ P(B).

(b) (4 marks) Let R be a relation on the set A = {1, 2, 3} defined below R = {(1, 1),(2, 2),(3, 3),(1, 2),(2, 1),(1, 3),(3, 1)}.

Then R is an equivalence relation.

(c) (4 marks) There is no smallest positive rational number.

(d) (4 marks) Let An = (0,1n) and Bn = (−1n, 0) be open intervals for each n ∈ N. Then

(e) (4 marks) Let f, g, h be three functions from R to R. Then f ◦(g +h) = f ◦g +f ◦h.

(f) (4 marks) Suppose n ∈ N, n ≥ 2, and [a], [b], [c] ∈ Zn such that [c] 6= [0]. If [a] · [c] = [b] · [c], then [a] = [b].

(g) (4 marks) Let a, b, c ∈ R and c ≥ 0. If ab < c,

(h) (4 marks) Let P, Q, R and S be statements. Suppose that P is false and (R ⇒ S) ⇔ (P ∧ Q) is true. Find the truth values of R and S.

2. Show for all integers n ≥ 0 that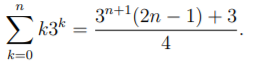

1. Prove: For all integers n we have 5 - n² − 2.




2. Prove or disprove: for every a, n ∈ N with n ≥ 2, there exist distinct k, ∈ N such that n divides ak − a.




3. Let A, B, C be sets. Prove: A × C ⊆ B × C if and only if A ⊆ B or C = ∅.




4. Suppose that S ⊆ R is a set defined by

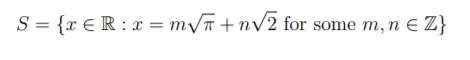
and S ' is a proper subset of S defined by

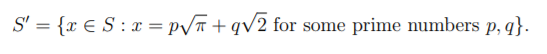
(a) Show that S is countably infinite.
(b) Is there a bijection from S' to S? Justify your answer.
(If needed, you may use the fact that π, √2  are irrational numbers without proof.)

1. Let x ∈ R satisfy 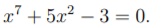 Prove that x is irrational.




2. Let f : A → B be a function. Prove:
   (a) (4 marks) If there is a function g : B → A such that g ◦ f(x) = x, for all x ∈ A, then f is injective.
   (b) (6 marks) If f is injective, then there is a function g : B → A such that g ◦ f(x) = x, for all x ∈ A.

This page has been left blank for your workings and solutions.

更多代写： HomeWork cs作业     金融代考    postgreSQL代写         IT assignment代写     统计代写 留学经验积累分享