ECE5606 Stochastic Signals and Systems:
随机信号代写 where x and y are stochastic processes and A, B, a and b are deterministic matrices and vectors, respectively.Is the process stationary
Consider the moving average process of order one x(t) = e(t) + ce(t − 1) where e(t) t = . . . , −1, 0, 1, . . . } is a sequence of independent normal (0, 1) random variables. 随机信号代写
(a) Determine the covariance of x(t) and x(s).
(b) Is the process stationary, normal, Markovian, ergodic, singular?
(c) Does the process have the independent increments?
Prove that
cov[Ax + a, By + b] = A{cov[x, y]}B T ,
where x and y are stochastic processes and A, B, a and b are deterministic matrices and vectors, respectively.
Consider the singular stochastic process {x(t), 0 ≤ t < ∞} defifined by 随机信号代写
where the initial state is normal (0, 1). Is the process ergodic? Give a predictor for the process which predicts x(t + h) based on measurements of x(t).
Consider the stochastic process
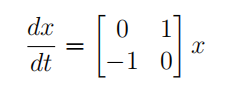
wheretheinitialstateisnormalwithzeromeanvalueandthecov ariance
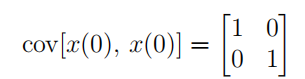
Is the process ergodic? Give a predictor for the process which predicts x(t + h) based on observation of {x1(t), t0 ≤ s ≤ t}.
Hint: The solution of the state of the system is given by
x(t) = Φ(t, 0)x(0),
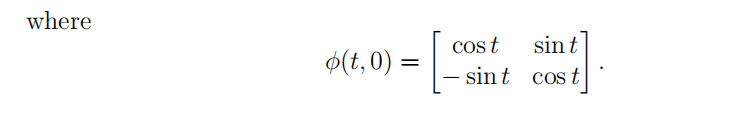

更多代写:cs代写 经济代考 会计代写 计算机科学代写 投资和金融市场法代写


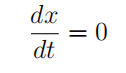


发表回复
要发表评论,您必须先登录。