Economics 3382A-001
Department of Economics
Gregory Pavlov Fall 2021
经济考试代考 What are the (pure strategy) Nash equilibria of each stage game? Now consider the two-stage game and suppose the payo§s of each player
Problem 1 (Technology adoption) 经济考试代考
During the adoption of the new technology a CEO (player 1) can design a new task for a division manager. The new task can be either high level (H) or low level (L). The manager simultaneously chooses to invest in good training (G) or bad training (B). The payo§s from this interaction are given by the following matrix:
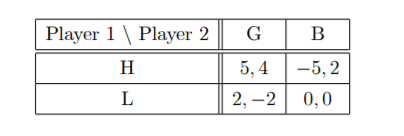
(a) Present the game in extensive form (a game tree) and solve for all (pure strategy) Nash equilibria and subgame perfect equilibria.
(b) Now assume that before the game is played the CEO can choose not to adopt this new technology, in which case the payo§s are (1,1), or to adopt it, in which case the game is played. Present the entire game in extensive form. How many proper subgames does it have?
(c) Solve for all (pure strategy) Nash equilibria and subgame perfect equilibria of the game described in (b).
Problem 2 (Campaigning revisited)
Two political candidates are scheduled to campaign in two states, in one in period t = 1 and in the other in t = 2. In each state each candidate can choose either a positive campaign that promotes his own agenda (P for player 1, p for player 2) or a negative one that attacks his opponent (N for player 1, n for player 2). Residents of the Örst-period state do not mind negative campaigns, which are generally e§ective, and payo§s in this state are given by the following matrix 经济考试代考
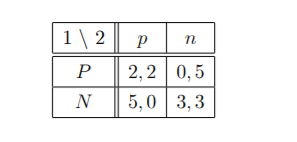
In the second-period state, residents dislike negative campaigns despite their e§ectiveness, and the payo§s are given by the following matrix:
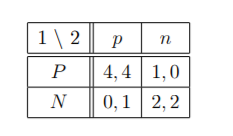
(a) What are the (pure strategy) Nash equilibria of each stage game? Now consider the two-stage game and suppose the payo§s of each player is the sum of the payo§s from each stage.
(b) Find all (pure strategy) subgame perfect equilibria in which the players choose (P, p) in the Örst stage.
(c) Find all (pure strategy) subgame perfect equilibria in which the players choose (N, n) in the Örst stage.
(d) Can you Önd a (pure strategy) subgame perfect equilibrium for the this game in which the players play (P, n) in the Örst stage?
Problem 3 (Timing claims on an investment) 经济考试代考
An amount of money accumulates over time; in period t; where t = 1; 2; 3, its size is $2t. In each period two people simultaneously decide whether to claim the money. If only one person does so, she gets all the money; if both people do so, they split the money equally; if neither person does so, both people have the opportunity to do so in the next period. If neither person claims the money in period 3, each person obtains $3. Each person only cares about the amount of money she obtains.
(a) Present the game in extensive form (game tree).
(b) Find all (pure strategy) subgame perfect equilibria.
 更多代写: HomeWork cs作业 金融代考 postgreSQL代写 IT assignment代写 统计代写 高级金融数学作业代写
更多代写: HomeWork cs作业 金融代考 postgreSQL代写 IT assignment代写 统计代写 高级金融数学作业代写




发表回复
要发表评论,您必须先登录。